MATHEMATICS

  TYPES OF TRIANGLE  TRIANGLE:  A triangle is a polygon with three edges and three                                vertices.                   It is one of the basic shapes in geometry.                     A triangle with vertices A,B and C is denoted. TYPES OF TRIANGLE: AREA OF TRIANGLE:

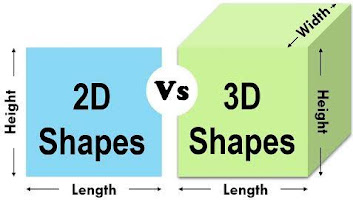
2D AND 3D SHAPES SHAPES:  The shape can be defined as the boundary or outline of an object. In general, we can see shapes such as triangles, squares, and circles everywhere around us. Thus shapes have only lenght and breadth are 2D or two-dimensional. while other shapes such as the shape of a house have length, breadth and height. Thus shapes are 3D or three-dimensional. 2D SHAPES:                           A 2D shapes has two dimensions lenght and breadth. examples: Rectangle, Square, Circle, Triangle. 3D SHAPES:                        A 2D shapes has three dimensions lenght, breadth and height. examples: Cuboid, Cube, Cylinder, Sphere. See the above image the 1st one is circle and it is in 2D shape and the 2nd one is sphere and it is in 3D shape. Here are some 2d and 3d shapes Differences between 2D and 3D shapes You (or the children in your class) might be wondering about the differences between 2D and 3D shapes. This is a really good question, since it can be confusing to understan

  CONCENTRIC AND CONGRUENT CIRCLES CIRCLE: A circle is the set of points in a plane that are equidistant from a given point. CONCENTRIC CIRCLE: Two or more circles that have the same center,but different radii are known as concentric circles. Concentric Circle Examples In Real Life Have you ever observed the wheel of a ship? It has two concentric circles joined by the spokes. Many of us have played darts. A dartboard also has concentric circles. The circles in the dartboard are drawn around a common center, that is, the bull's eye. CONGRUENT CIRCLE: Two or more circles with the same radius,but different centres are known as congruent circles.